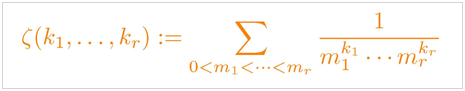
Multiple zeta values and functions are defined by the following nested infinite series.
When the arguments are all positive integers, this is called the multiple zeta value; on the other hand, as a function of complex variables, this is called the multiple zeta function. When the number of variables is one, this is nothing but the celebrated Riemann zeta function. In this study, we use the general term “multiple zetas” to refer not only to these values and functions but also to more generalized versions of those. Euler initiated the study of these objects more than two centuries ago, but it is since only about two decades that active research has been conducted in connection with a variety of areas in mathematics as well as mathematical physics.
During the period of our research project, we attempt to reveal connections between various results and conjectures on multiple zeta values derived since the past two decades, and try to find a unified viewpoint, which may probably still be hidden. Further, we develop an analytic and p-adic theory of multiple zeta functions, and with the theory of multiple zeta values, we attempt to contribute a new development in the area of multiple zetas.